Color 3-D Prints

Color Studies of Enneper's Minimal Surface

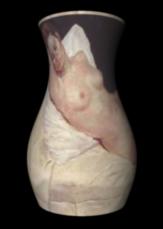
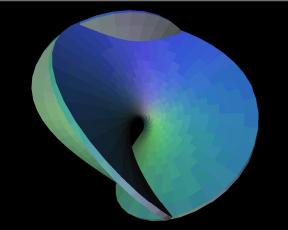
The Gauss Map of a surface associates to each
point on the surface its unit
normal vector.

In these studies, the Unit Normals
to the surface are used to create a color mapping on the surface
which imitates the default Mathematica
(lighting)/rendering model.

LightSources
  {{{1.,0.,1.},RGBColor[1,0,0]},{{1.,1.,1.},
      RGBColor[0,1,0]},{{0.,1.,1.},RGBColor[0,0,1]}}

In these studies, however, the color calculations are done in CMY color space and the background is white.

Stewart Dickson has written the software to convert the Gauss-map of an object to a Mathematica-style surface color map. Download here.

Color Snakeskin Texture-Mapped Trefoil Torus Knot

The texture image is parametrically
mapped along the knotted torus.

Color 3-D Printing was done by Z Corporation. Download here.

Logarithmic Spiral Snail

A snail shell generated in Mathematica Download here.

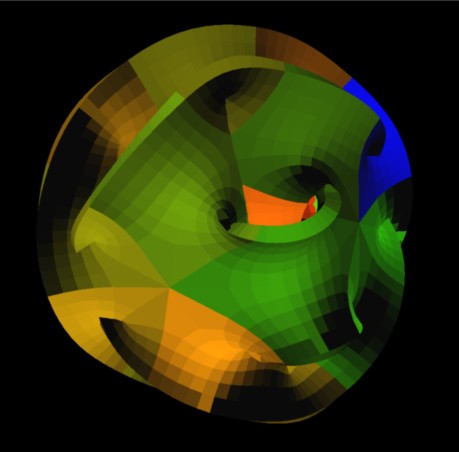The Seventeenth-Century French mathematician Pierre de Fermat wrote in the margin of his copy of Arithmetica by Diophantus, near the section on the Pythagorean Theorem (a squared plus b squared equals c squared),

"x ^ n + y ^ n = z ^ n - it cannot be solved with non-zero integers x, y, z for any exponent n greater than 2. I have found a truly marvelous proof, which this margin is too small to contain."

This was left as an enigmatic riddle after Fermat's death and it became a famous, unsolved problem of number theory for over 350 years.

Andrew Hanson has made some pictures, and I have in turn made sculpture, of a system analogous to Fermat's last theorem - a superquadric surface parameterized in complex four-space.

We think that the mathematics of the n=3 case are similar to Fermat's own proof of the n=3 special case. Our pictures have lent some visual concreteness to the recent news of Andrew Wiles' proof of the Taniyama-Weil conjecture, which implies the proof of Fermat.

3-D Fractals

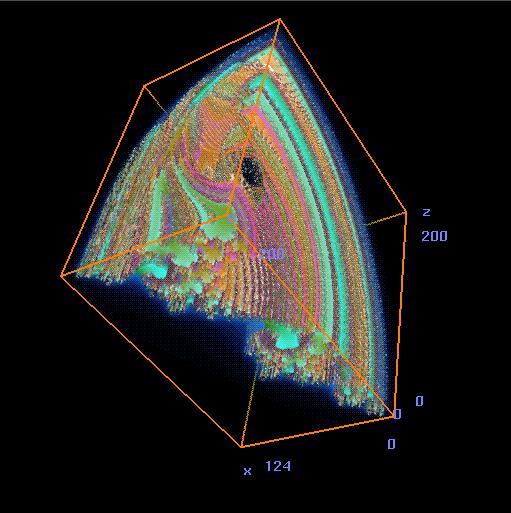The Z Corporation 3-D Color Printer has a Bit-Mapped, color image slice-based software interface to its build process. This interface makes the Z Corp machine the ideal platform on which to execute the Fractal Zoom in Three Physical Dimensions -- and in color!

Stewart Dickson has written the software to interface this data object to the Z Corp 3-D Color Printer. Download here.

Painted Vases

The artist has developed the
software to generate a Vase,
as a mathematical function,
and to map a color digital
image onto the surface as a
VRML V2.0 file.

This file format is suitable
for output using the Z Corp
Color 3-D Printer. Download here.

"Botty Shelly"

The Surreal Thing

Newell's "Utah Teapot" as prepared for 3D printing with a Magritte and J. Turner Whitted-inspired faux environment mapped to the surface.

Please take a moment to complete our Reader's Response Form.
Or contact:

Stewart Dickson, Sculptor
110 N. Whipple St.
Fort Bragg, CA  95437 USA
(707)813-0385
MathArtSPD@gmail.com

Press Here to go to Mathart.org home page.